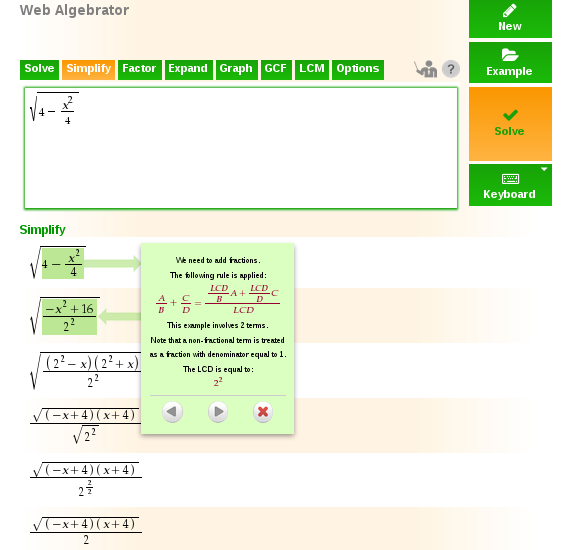
The problem
Answer provided by our tutors
Clicking on a particular solution can allow you to review the logic which is employed. I would always encourage users to click on the 'Options' tab and select 'a few steps' initially and only increase the number of solution steps when the extra information is useful and clarifying.
So the first step to this simplification is a least common denominator for adding "4-(x^2)/4" and the common denominator is '4' which is going to be expressed as 2^2. The representation of a number by its prime factorization is standard with the software and we do realize that it can create some awkwardness. Please understand that the use of prime factorization does ensure that cancellations are properly recognized and its use can be viewed as a "best practice". For example, when working with fractions it is not immediately obvious that "91/28" has any cancellations at all, while the prime-factored version, "(7 * 13) / (2*2 * 7)", has an obvious cancellation of sevens.
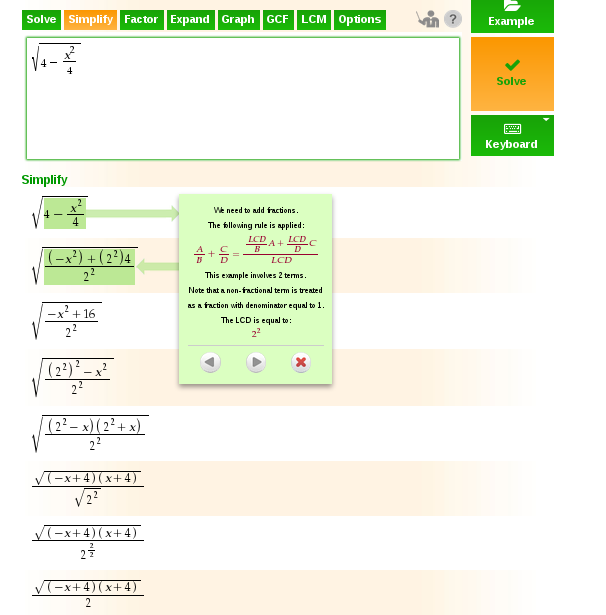
So the same simplification using 'a few steps':